Sudoku Puzzle #86 & #87

Dan LeKander -

Can you believe it is the middle of July!   Pick a rainy morning and give yourself a hearty  Sudoku challenge.

Clueless?


As a bonus each month this year we will start with a Sudoku puzzle in progress, where it appears there are no more obvious or not-so-obvious clues.  Does this puzzle #86 have any more clues.

Puzzle #86

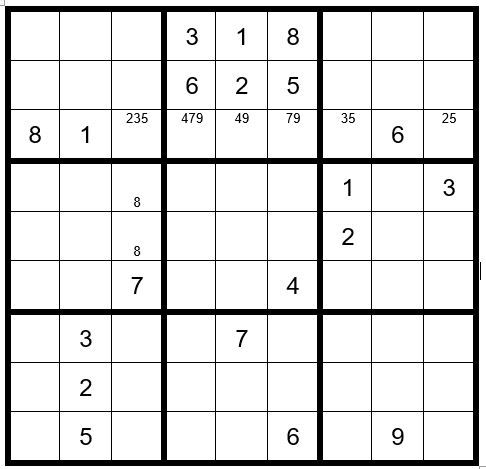
(The answer follows below after the conclusion of Puzzle #87, the feature puzzle for July)

Feature Puzzle


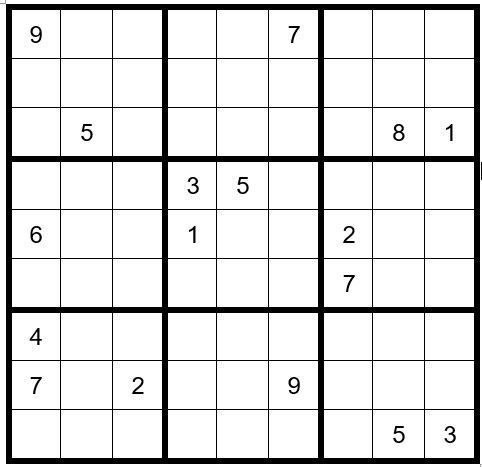
The “Impossible” Series continues with Puzzle #87, the feature puzzle for July …

Puzzle #87

DAN’S 8-STEP APPROACH TO SOLVING ALL SUDOKU PUZZLES

Once you have  completed the puzzle, to the extent that you have filled-in all obvious  answers and have written all potential options across the top of the  unsolved cells (PUZZLE PREPARATION), Dan recommends the following Steps  to complete the puzzle.

Step 1:  Sudoku Pairs, Triplets and Quads – See September 2015
Step 2:  Turbos & Interaction – See October 2015
Step 3:  Sudoku Gordonian Rectangles and Polygons – See November 2015
Step 4:  XY-Wings & XYZ Wings – See December 2015
Step 5:  X-Wings – See January 2016

Step 6:  DAN’S YES/NO CHALLENGE
Step 7:  DAN’S CLOSE RELATIONSHIP CHALLENGE
Step 8:  AN EXPANSION OF STEP 7Steps  1-5 are relatively common techniques and are explained in the TI LIFE  articles above. Steps 6-8 are covered in detail, in Dan’s book.

PUZZLE PREPARATION

Prior to utilizing Steps 1-8, complete the 5 Steps of Puzzle Preparation …

  1. FILL IN DATA FROM OBSERVATIONS
  2. FILL IN OBVIOUS ANSWERS
  3. FILL IN NOT-SO-OBVIOUS ANSWERS
  4. MARK UNSOLVED CELLS WITH OPTIONS THAT CANNOT EXIST IN THOSE CELLS
  5. FILL IN THE OPTIONS FOR THE UNSOLVED CELLS

We will complete all of the first 4 steps in the order we observe them, until we conclude all Puzzle Preparation Step 1-4 clues.

The first thing we observe is that C8R7 & C9R7 have options 27.

Next, C3R7=5.  And C1R6=5, C9R5=5, C7R1=5, C4R8=5 & C6R2=5.  This concludes the 5’s.

In box three a 7 can only exist as an option in C8R2 or C9R2; therefore, C2R2 & C3R3 cannot have a 7 as an option.  

Now the only cell in box 1 that can be a 7 is C3R3.  C3R3=7.  Then C5R5=7.  

The options 27 in C8R7 & C9R7 prevent C4R7, C5R7 & C6R7 from having a 7 as an option; therefore, C4R9=7.  C2R4=7

Now your grid should look like Example #87.1 below:

Example #87.1

Look at box 2.  In box 2 a 1 can only exist as an option in C5R1 or C5R2; therefore, a 1 cannot exist as an option in C5R7, C5R8 and C5R9.

In box 6 a 3 can only exist as an option in C8R5 or C8R6; therefore, a 3 cannot exist as an option in C8R1 or C8R2.
In box 7 a 3 can only exist as an option in C2R7 or C2R8; therefore, a 3 cannot exist as an option in C2R1, C2R2, C2R5 or C2R6.  

Now you can see that in box 4 a 3 can only exist as an option is C3R5 or C3R6; therefore, a 3 cannot exist as an option in C3R1 or C3R2.

In box 5 a 9 can only exist as an option in C4R6 or C5R6; therefore, a 9 cannot exist as an option in C2R6, C3R6, C8R6 or C9R6.

In box 9 a 9 can only exist as an option in C7R7 or C7R9; therefore a 9 cannot exist as an option in C7R2, C7R3 or C7R4.

Now your grid should look like Example #87.2 below:

Example #87.2

What do you notice about row 1?  

The only cell that can be a 3 is C5R1.  C5R1=3.  Then, C6R7=3 and C2R8=3.  Now C5R2=1, C6R9=1, C2R7=1, C3R1=, C1R4=1 C8R6=1, and C7R8=1.

From this point the puzzle is easily solved without advanced techniques, leading to a solution per Example 87.3 below:

Example #87.3

The first time I tackled this puzzle I missed the clue of C5R1=3.  I solved the puzzle with a Step 7 exercise.  This is just one of many, many examples of how a Sudoku puzzle can be solved in different ways.

May the gentle winds of Sudoku be at your back.

Dan LeKander

Puzzle #86

Clue for Puzzle #86 … focus your attention on row 6.   What do you observe?

There are only two unsolved cells in row 6 that can have options 12, which are C1R6 and C4R6.  

Now the only unsolved cell in row 6 that can be a 3 is C5R6.   C5R6=3.

This leads to C6R8=3, as well as additional answers.

Editor's Note:  Summer 2021!

Dan and his proofreader (and, as they say, better half) Peggy, give us a new challenge each month.

It was back in January 2016, when we published a final article in Dan's Series of Steps to learn the logic of Sudoku –  he asked if we would like a puzzle to solve every month … this editor said an enthusiastic… Yes, please!

If you have not already done so, I suggest you purchase Dan’s book: “3 Advanced Sudoku Techniques, That Will Change Your Game Forever!” Purchase of a book includes a 50-page blank grid pad, 33 black and two green tokens, to assist with Step 6.…

I also encourage you to write to Dan and tell him how his system is helpful!

The book is available online at ebay.com.

Be sure to read the TI Life's review of Dan's book by Jesse Kahn published in Jun 2015.

Here are links to all past Sudoku Puzzle Challenge beginning: February 2016, March 2016, April 2016, May 2016, June 2016, July 2016, August 2016, September 2016, October 2016, November 2016, December 2016, January 2017, February 2017, March 2017, April 2017, May 2017, June 2017, July 2017, August 2017, September 2017, October 2017, November 2017, December 2017, January 2018, February 2018, March 2018, April 2018, May 2018, June 2018, July 2018, August 2018, September 2018, October 2018, November 2018, December 2018, January 2019, February 2019, March 2019, April 2019, May 2019, June 2019, July 2019, August 2019, September 2019, October 2019, November 2019, December 2019, January 2020, February 2020, April 2020, May 2020,  June 2020 and July 2020, August 2020,  September 2020, October 2020, November 2020 and December 2020, January 2021, February 2021, March 2021, April 2021, May 2021 and June 2021.